Gamma - The Rubyist Blog

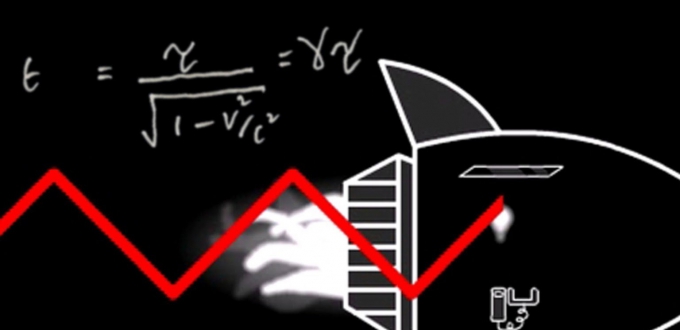
While I was watching a video from one of my favorite YouTube channels, I decided I wanted to try the math(s) that Professor Merrifield was describing to explain Gamma / the Lorentz factor. I’m very excited that my new blog supports $\LaTeX$ and MathJax so the formulas actually turn out looking the way they should.

While this math might look difficult at first, it is really easy. It is essentially just using the Pythagorean theorem to isolate the relationship between $t$ and $\tau$ , which is the factor by which time (and length, and mass) changes for a moving object.

So, without further ado:

Calculating $\gamma$ (the Lorentz factor):

Where:

$v$ is the relative velocity between inertial reference frames (the velocity of the moving observer)
$\tau$ is the proper time for a moving observer (time from the moving observers own perspective)
$t$ is the time as observed by a stationary observer
$c$ is the speed of light in a vacuum

This is much easier to understand by drawing out the right triangle, just like Professor Merrifield did in the video:

Based on that, we can start with this by squaring the sides:
$(tc)^2 = (\tau c)^2 + (tv)^2$

Rewriting it this way will make it easier to move things around:
$t^2c^2 = \tau^2c^2 + t^2v^2$

Subtract $t^2v^2$ from both sides:
$t^2c^2 - t^2v^2 = \tau^2c^2$

Divide everything by $c^2$ :
$t^2 - t^2\frac{v^2}{c^2} = \tau^2$

Factor out $t^2$ :
$t^2\left(1-\frac{v^2}{c^2}\right) = \tau^2$

Isolate $t^2$ :
$t^2 = \frac{\tau^2}{1-\frac{v^2}{c^2}}$

Take the principal square root of both sides:
$t = \frac{\tau}{\sqrt{1-\frac{v^2}{c^2}}}$

Factor out $\tau$ on the right:
$t = \tau\left(\frac{1}{\sqrt{1-\frac{v^2}{c^2}}}\right)$

And now we have gamma, as the relationship between $t$ and $\tau$ :
$t = \tau\gamma$

Thus we have defined gamma like so:
$\gamma = \frac{1}{\sqrt{1-\frac{v^2}{c^2}}}$

I obviously can’t take credit for all the math here, as I had a lot of help from the sixty symbols video. Those guys are great. I highly encourage everyone to check out their site: http://www.sixtysymbols.com/.

If you have any questions about the math in this post, feel free to leave a comment. In case you missed it in the text above, here’s another link to the video about Gamma: https://youtu.be/Cxqjyl74iu4

Spread the love

Like this: